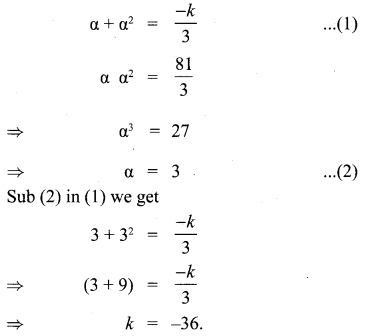You can Download Samacheer Kalvi 10th Maths Book Solutions Guide Pdf, Tamilnadu State Board help you to revise the complete Syllabus and score more marks in your examinations.
Tamilnadu Samacheer Kalvi 10th Maths Solutions Chapter 3 Algebra Ex 3.14
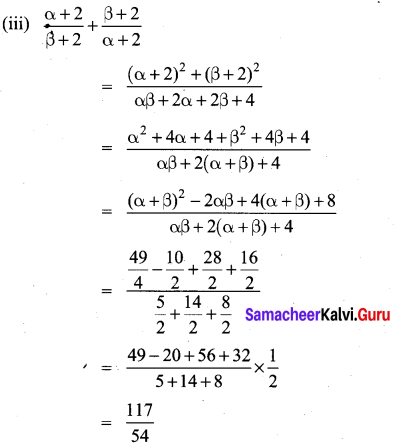
10th Maths Exercise 3.14 Solutions Question 1.
Write each of the following expression in terms of α + β and αβ.
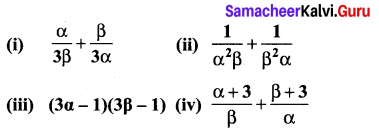
Solution:

Ex 3.14 Class 10 Samacheer Question 2.
The roots of the equation 2x2 – 7x + 5 = 0 are α and β. Without solving the root find
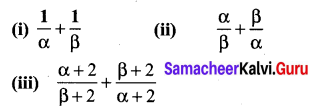
Solution:
2x2 – 7x + 5 = x2 – \(\frac{7}{2} x+\frac{5}{2}\) = 0
α + β = \(\frac{7}{2}\)
αβ = \(\frac{5}{2}\)
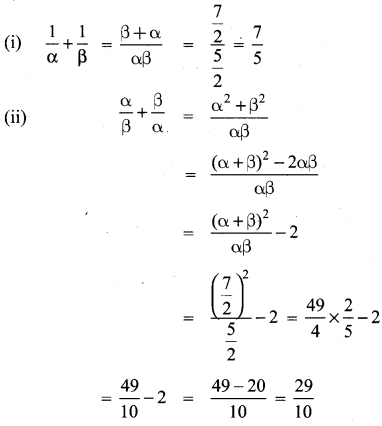
10th Maths Exercise 3.14 Question 3.
The roots of the equation x2 + 6x – 4 = 0 are α, β. Find the quadratic equation whose roots are
(i) α2 and β2
(ii) \(\frac{2}{\alpha} \text { and } \frac{2}{\beta}\)
(iii) α2β and β2α
Solution:
If the roots are given, the quadratic equation is x2 – (sum of the roots) x + product the roots = 0.
For the given equation.
x2 + 6x – 4 = 0
α + β = -6
αβ = -4
(i) α2 + β2 = (α + β)2 – 2αβ
= (-6)2 – 2(-4) = 36 + 8 = 44
α2β2 = (αβ)2 = (-4)2 = 16
∴ The required equation is x2 – 44x – 16 = 0.
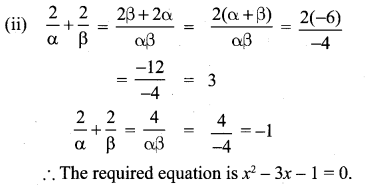
(iii) α2β + β2α = αβ(α + β)
= -4(-6) = 24
α2β × β2α = α3β3 = (αβ)3 = (-4)3 = -64
∴ The required equation = x2 – 24x – 64 – 0.
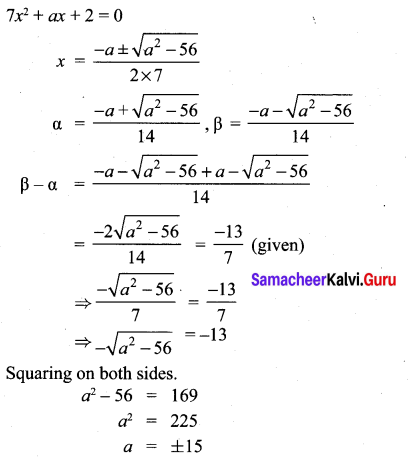
10th Maths Exercise 3.14 Samacheer Kalvi Question 4.
If α, β are the roots of 7x2 + ax + 2 = 0 and if β – α = \(\frac{-13}{7}\) Find the values of a.
Solution:
10th Maths Exercise 3.14 Solution Question 5.
If one root of the equation 2y2 – ay + 64 = 0 is twice the other then find the values of a.
Solution:
Let one of the root α = 2β
α + β = 2β + β = 3β
Given
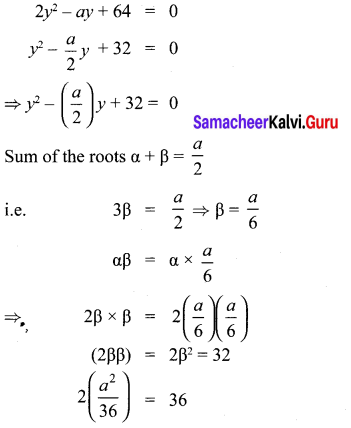
a2 = 576
a = 24, -24
Exercise 3.14 Class 10 Samacheer Question 6.
If one root of the equation 3x2 + kx + 81 = 0 (having real roots) is the square of the other then find k.
Solution:
3x2 + kx + 81 = 0
Let the roots be α and α2