Samacheer Kalvi 11th Maths Solutions Chapter 5 Binomial Theorem, Sequences and Series Ex 5.2

You can Download Samacheer Kalvi 11th Maths Book Solutions Guide Pdf, Tamilnadu State Board help you to revise the complete Syllabus and score more marks in your examinations.

Tamilnadu Samacheer Kalvi 11th Maths Solutions Chapter 5 Binomial Theorem, Sequences and Series Ex 5.2

Question 1.
Write the first 6 terms of the sequences whose n^th terms are given below and classify them as arithmetic progression, geometric progression, arithmetico-geometric progression, harmonic progression and none of them.

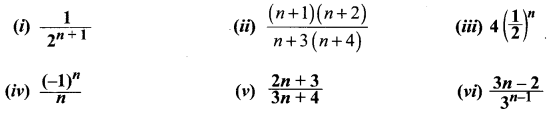
Solution:


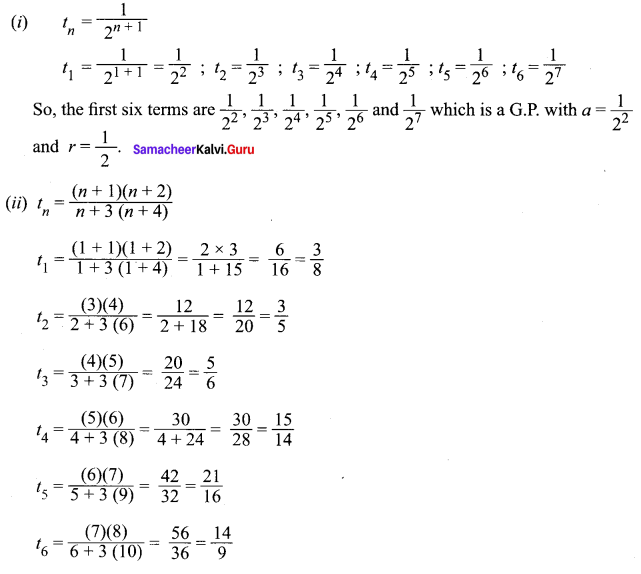
It is not a G.P. or A.P. or H.P. or A.G.P.

It is not an A.P. or G.P. or H.P. or A.G.P

It is not an A.P. or G.P. or H.P. or A.G.P.


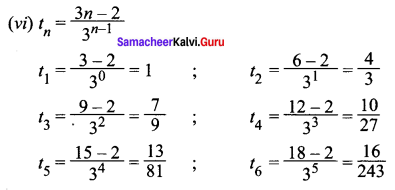
It is a A.G.P.

Question 2.
Write the first 6 terms of the sequences whose n^th term a_n is given below.

Solution:
a₁ = 1 + 1 = 2 ; a₂ = 2
a₃ = 3 + 1 = 4 ; a₄ = 4
a₅ = 5 + 1 = 6 ; a₆ = 6
So, the first 6 terms are 2, 2, 4, 4, 6, 6

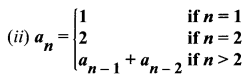
Solution:
a₁ = 1 ; a₂ = 2, a₃ = 3
a₄ = a₃ + a₂ + a₁ = 3 + 2 + 1 = 6 ⇒ a₄ = 6
a₅ = a₄ + a₃ + a₂ = 6 + 3 + 2 = 11 ⇒ a₅ = 11
a₆ = a₅ + a₄ + a₃ = 11 + 6 + 3 = 20 ⇒ a₆ = 20
So the first 6 terms are 1, 2, 3, 5, 8, 13.

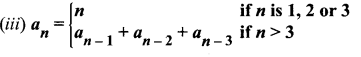
Solution:

Question 3.
Write the n_th term of the following sequences.
Solution:
(i) 2, 2, 4, 4, 6, 6……
Solution:

Solution:
Nr: 1, 2, 3, ……t_n = n
Dr: 2, 3, 4, …..t_n = n + 1


Solution:
Nr: 1, 3, 5, 7, . . .which is an A.P. a = 1, d = 3 – 1 = 2
t_n = a + (n – 1)d
t_n = 1 + (n – 1)2 = 1 + 2n – 2 = 2n – 1.
Dr : 2, 4, 6, 8, . . .
So the n^th term is 2 + (n – 1)2 = 2 + 2n – 2 = 2n.

(iv) 6, 10, 4, 12, 2, 14, 0, 16, -2,….
Solution:
t₁ = 6 ; t₂ = 10
t₃ = 4 ; t₄ = 12
t₅ = 2 ; t₆ = 14
t₇ = 0 ; t₈ = 16
When n is odd, the sequence is 6, 4, 2, 0,…
(i.e.) a = 6 and d = 4 – 6 = -2.
So, t_n = 6 + (n – 1)(-2) = 6 – 2n + 2 = 8 – 2n
When n is even, the sequence is 10, 12, 14, 16,…
Here a = 10 and d = 12 – 10 = 2
t_n = 10 + (n – 1)2 = 10 + 2n – 2 = 2n + 8 (i.e.) 8 + 2n

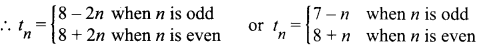
Question 4.
The product of three increasing numbers in GP is 5832. If we add 6 to the second number and 9 to the third number, then resulting numbers form an AP. Find the numbers in GP.
Solution:
The 3 numbers in a G.P. is taken as \(\frac{a}{r}\), a, ar
Their product is 5832.

6r² + 6 = 13
6r² – 13r + 6 = 0
(3r – 2)(2r – 3) = 0
r = 2/3 or 3/2

Question 5.

Solution:

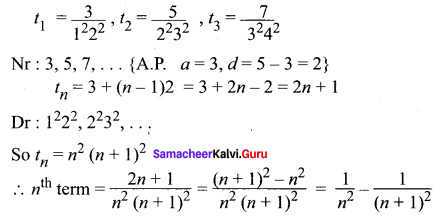
Question 6.
If t_k is the k^th term of a G.P., then show that t_{n – k}, t_n, t_{n + k} also form a GP for any positive integer k.
Solution:
Let a be the first term and r be the common ratio.
We are given t_k = ar^{k – 1}
We have to Prove : t_{n – k}, t_n, t_{n + k} form a G.P.

Question 7.
If a, b, c are in geometric progression, and if \(a^{\frac{1}{x}}=b^{\frac{1}{y}}=c^{\frac{1}{z}}\), then prove that x, y, z are in arithmetic progression.
Solution:
Given a, b, c are in G.P.
⇒ b² = ac
⇒ log b² = log ac
(i.e.) 2log b = log a + log c …(1)

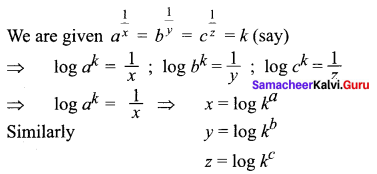
Substituting these values in equation (1) we get 2y = x + z ⇒ x, y z are in A.P.

Question 8.
The AM of two numbers exceeds their GM by 10 and HM by 16. Find the numbers.
Solution:
Let the two numbers be a and b.

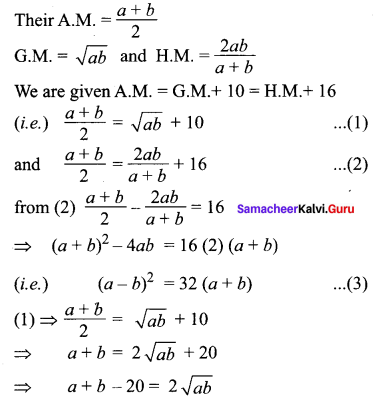
So, (a + b – 20)² = 4ab
(i.e.) (a + b)² + 400 – 40(a + b) = 4ab
(a + b)² – 4ab = 40(a + b) – 400
from(3) (a + b)² – 4ab = 32(a + b)
⇒ 32(a + b) = 40(a + b) – 400
(÷ by 8) 4(a + b) = 5(a + b) – 50
4a + 4b = 5a + 5b – 50
a + b = 50
a = 50 – b
Substituting a = 50 – b in (3) we get
(50 – b – b)² = 32(50)
(50 – 2b)² = 32 × 50

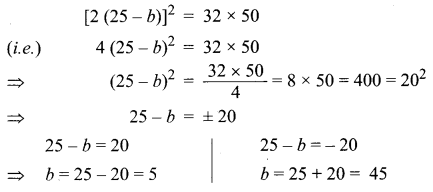
When b = 5, a = 50 – 5 = 45
When b = 45, a = 50 – 45 = 5
So the two numbers are 5 and 45.

Question 9.
If the roots of the equation (q – r)x² + (r – p)x + p – q = 0 are equal, then show that p, q and r are in AP.
Solution:
The roots are equal ⇒ ∆ = 0
(i.e.) b² – 4ac = 0
Hence, a = q – r ; b = r – p ; c = p – q
b² – 4ac = 0
⇒ (r – p)² – 4(q – r)(p – q) = 0
r² + p² – 2pr – 4[qr – q² – pr + pq] = 0
r² + p² – 2pr – 4qr + 4q² + 4pr – 4pq = 0
(i.e.) p² + 4q² + r² – 4pq – 4qr + 2pr = 0
(i.e.) (p – 2q + r)² = 0
⇒ p – 2q + r = 0
⇒ p + r = 2q
⇒ p, q, r are in A.P.

Question 10.
If a, b, c are respectively the p^th, q^th and r^th terms of a G.P., show that (q – r) log a + (r – p) log b + (p – q) log c = 0.
Solution:
Let the G.P. be l, lk, lk²,…
We are given t_p = a, t_q = b, t_r = c

LHS = (q – r) log a + (r – p) log b + (p – q) log c

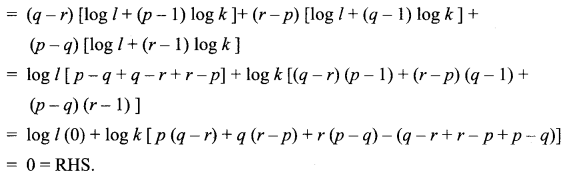
Samacheer Kalvi 11th Maths Solutions Chapter 5 Binomial Theorem, Sequences and Series Ex 5.2 Additional Questions Solved

Question 1.

Solution:
Here a₁ = 1
Substituting n = 2, we obtain a₂ = a₁ + 2 = 1 + 2 = 3
Substituting n = 3, 4 and 5, we obtain respectively
a₃ = a₂ + 2 = 3 + 2 = 5, a₄ = a₃ + 2 = 5 + 2 = 7
a₅ = a₄ + 2 = 7 + 2 = 9
Thus, the first five terms are 1, 3, 5, 7 and 9.

Question 2.
Find the 18^th and 25^th term of the sequence defined by

Solution:
When n = 18 (even)
a_n = n(n + 2) = 18(18 + 2) = 18(20) = 360
When n = 25(odd)

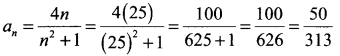
Question 3.
Write the first six terms of the sequences given by
(i) a₁ = a₂= 1 = a_{n – 1} + a_{n – 2} (n ≥ 3)
(ii) a₁ = 4, a_{n + 1} = 2na_n
Solution:
(i) Here a₁ = a₂= 1 = a_{n – 1} + a_{n – 2} (n ≥ 3)
Putting n = 3, a₃= a₂ + a₁ = 1 + 1 = 2
Putting n = 4, a₄ = a₃ + a₂ = 2 + 1 = 3
Putting n = 5, a₅ = a₄ + a₂ = 3 + 2 = 5
Putting n = 6, a₆ = a₅ + a₄ = 5 + 3 = 8
∴ First six terms of the sequence are 1, 1, 2, 3, 5, 8

(ii) Here a₁ = 4 and a_{n + 1} = 2na_n
Putting n = 1, a₂ = 2 × 1 × a₁ = 2 × 1 × 4 = 8
Putting n = 2, a₃ = 2 × 2 × a₂ = 4 × 8 = 32
Putting n = 3, a₄ = 8 × 192 = 1536
Putting n = 4, a₅ = 2 × 4 × a₄ = 8 × 192 = 1536
Putting n = 5, a₆ = 2 × 5 × a₅ = 10 × 1536 = 15360

Question 4.
An A.P. consists of 21 terms. The sum of the three terms in the middle is 129 and of the last three is 237. Find the series.
Solution:
Let a₁ be the first term and d, be the common difference. Here n = 21.
∴ The three middle terms are a₁₀,a₁₁, a₁₂
Now, a₁₀ + a₁₁ + a₁₂ = 129 [Given]
∴ (a₁ + 9d) + (a₁ + 10d) + (a₁ + 11d) = 129
⇒ 3a₁ + 30d = 129 ⇒ a₁ + 10d = 43 ……(i)
The last three terms are a₁₉, a₂₀, a₂₁
a₁₉, a₂₀, a₂₁ = 237 [Given]
∴ (a₁ + 18d)+(a₁ + 19d) + (a₁ + 20d) = 237
(i.e.,) 3a₁ + 57d = 237 ⇒ a₁ + 19d = 79 … (2)
Subtracting (i) from (ii), we get 9d = 36, ⇒ d = 4
∴ From (i), a₁ + 40 = 43 ⇒ a₁ = 3
Hence, the series is 3, 7, 11, 15 …….

Question 5.
Prove that the product of the 2^nd and 3^rd terms of an arithmetic progression exceeds the product of the first and fourth by twice the square of the difference between the 1^st and 2^nd.
Solution:
Let ‘a’ be the first term and ‘d’ be the common difference of A.P.
Then, a₁ = a, a₂ = a + (2 – 1)d = a + d
a₃ = a + (3 – 1)d = a + 2d, a₄ = a + (4 – 1)d = a + 3d
We have to show that a₂.a₃ – a₁.a₄ = 2(a₂ – a₁)²
LHS = a₂.a₃ – a₁.a₄ = (a + d)(a + 2d) – a(a + 3d)
= a₂ + 3ad + 2d₂ – a₂ – 3ad = 2d₂
RHS = 2(a₂ – a₁)² = 2d₂
Since LHS = RHS. Hence proved.

Question 6.
If the p^th, q^th and r^th terms of an A.P. are a, b, c respectively, prove that a(q – r) + b (r – p) + c(p – q) = 0.
Solution:
Let A be the first term and D be the common difference of A.P.
a_p = a, ∴ A + (p – 1)D = a ….. (1)
a_q = b, ∴ A + (q – 1)D = b ……. (2)
a_r = c, ∴ A + (r – 1)D = c …….. (3)
∴ a (q – r) + b (r – p) + c (p – q) = [A + (p – l) D] (q – r) + [A + (q – 1) D]
(r – p) + [A + (r – 1) D] (p – q) [Using (1), (2) and (3)]
= (q – r + r – p + p – q)A + [(p – l)(q – r) + (q – l)(r – p) + (r – l)(p – q)]D
= (0) A + (pq – pr – q + r + qr – pq – r + p + pr – p – qr + q)D
= (0)A + (0)D = 0.

Question 7.
If a, b, c are in A.P. and p is the A.M. between a and b and q is the A.M. between b and c, show that b is the A.M. between p and q.
Solution:
a, b, c are in A.P.
2b = a + c …… (1)
p is the A.M. between a and b

q is the A.M. between b and c


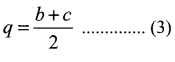
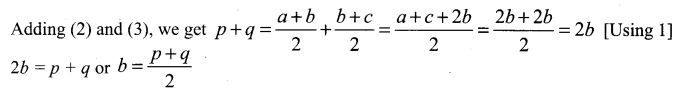
Hence, b is the A.M. between p and q

Question 8.
If x, y, z be respectively the p^th, q^th and r^th terms of a G.P. show that x^{q – r}, y^{r – p} ,z^{p – q} = 1
Solution:
Let A be the first term and R be the common ratio of G.P.
a_p = x ⇒ x = AR^{p – 1} ……… (1)
a_q = y ⇒ x = AR^{q – 1} ……… (2)
a_r = z ⇒ x = AR^{r – 1} ……… (3)
Raising (1), (2), (3) to the powers q – r, r – p, p – q respectively, we get

Multiplying (4), (5) and (6), we get

Hence, x^{q – r}, y^{r – p}, z^{p – q} = 1