Samacheer Kalvi 12th Maths Solutions Chapter 8 Differentials and Partial Derivatives Ex 8.5

You can Download Samacheer Kalvi 12th Maths Book Solutions Guide Pdf, Tamilnadu State Board help you to revise the complete Syllabus and score more marks in your examinations.

Tamilnadu Samacheer Kalvi 12th Maths Solutions Chapter 8 Differentials and Partial Derivatives Ex 8.5

Question 1.
If w(x, y) = x³ – 3xy + 2y², x, y ∈ R, find the linear approximation for w at (1, -1) .
Solution:
w(x, y) = x³ – 3xy + 2y² ; at (1, -1)
Linear approximation is given by

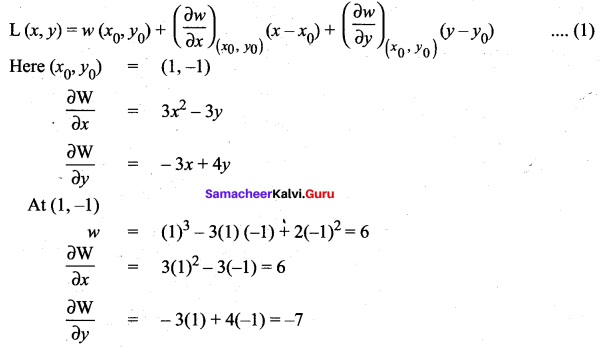
(1) ⇒ Let(x, y) = 6 + 6(x – 1) – 7(y + 1)
= 6x – 6 – 7y – 7
= = 6x – 7y – 7

Question 2.
Let z(x, y) = x²y + 3xy⁴, x, y ∈ R, Find the linear approximation for z at (2, -1).
Solution:

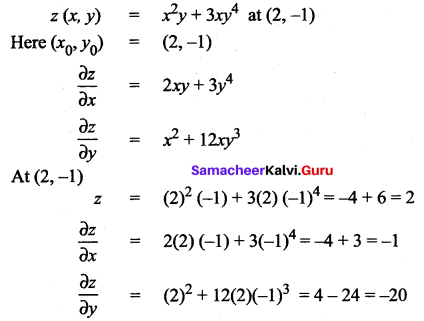
Linear approximation is given by

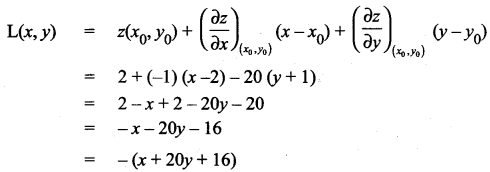
Question 3.
If v(x, y) = x² – xy + \(\frac{1}{4}\)y² + 7, x, y ∈ R, find the differential dv.
Solution:

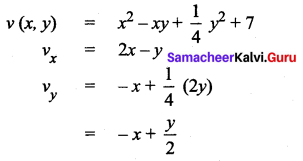
The differential is dv = (2x -y) dx + (- x + \(\frac{y}{2}\))dy

Question 4.
Let W(x, y, z) = x² – xy + 3sinz,x,y, z ∈ R. Find the linear approximation at (2, -1, 0).
Solution:
w (x, y, z) = x² – xy + 3 sin z
Here(x₀, y₀, y₀) = (2, -1, 0)

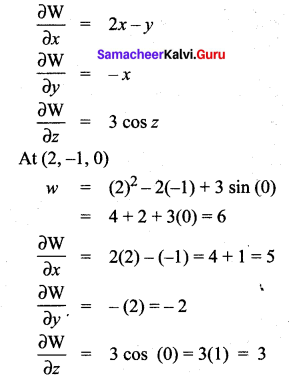
Linear approximation is given by

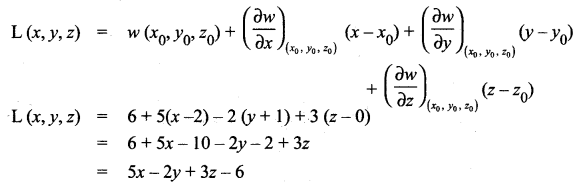
Question 5.
Let V (x, y, z) = xy + yz + zx, x, y, z ∈ R. Find the differential dV.
Solution:
V(x, y, z) = xy + yz + zx
V_x = y + z
V_y = x + z
V_z = y + x
The differential is dV = (y + z) dx + (x + z) dy + (y + x) dz